Week 7 Monday: Hyperbolic Groups and Quasi-Isometry

Hyperbolic Groups

The next thrust of the course is hyperbolic groups. We care about hyperbolic groups for four reasons:

In many models of “random groups,” groups are hyperbolic with probability 1. For example, under the few-relators model of random groups, where we pick relators at random given a set of generators, as the allowed length of relators goes to 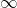$\infty$ , the probability of the group generated being hyperbolic goes to 1.
All free groups and finite groups are hyperbolic.
All hyperbolic groups are automatic.
Hyperbolic groups have an exceptionally nice solution to the word problem.

We will now give the definition of a hyperbolic group, acknowledging that there are two words that we have not previously defined.

Definition. A group $G$ is hyperbolic if it acts geometrically on a $\delta-$ hyperbolic space.

From the motivating facts above, one might think that it is very difficult to find groups that are not hyperbolic! This is in fact not the case. For exception, any group which contains $\mathbb{Z}^2$ (including $\mathbb{Z}^2$ itself) is not hyperbolic. Additionally, the Baumslag–Solitar groups are also not hyperbolic. We don’t have the tools yet to show that these are not hyperbolic, they just serve as examples to show that not every group we know about falls under this new categorization.

Next, we will discuss isometries and quasi-isometries, which we will need when thinking about $\delta-$ hyperbolic spaces.

Isometries

We begin by reviewing the definition of a metric space.

Definition. A metric space is a set $X$ along with a metric on $X$ , $d_x : X\times X \rightarrow \mathbb{R}_{\ge 0}$ such that the metric satisfies three conditions:

$d_x(x,y)=0$ if and only if $x=y$ ,
$d_x(x,y)=d_x(y,x)$ , and
$d_x(x,y) + d_x(y,z) \ge d_x(x,z)$ .

Then,

Definition. An isometry is a map from one metric space to another (or itself) that preserves distances. That is,

$f: X \rightarrow Y$ such that $d_x(x,y)=d_y(f(x),f(y))$ .

Additionally,

Definition. Two spaces, $X$ and $Y$ , are isometric if there exists a surjective isometry from $X$ to $Y$ .

Let us now consider a couple of examples. We know that $mathbb{E}^2$ ( $\mathbb{R}$ with the Euclidean metric) is a metric space. Isometries of $\mathbb{E}^2$ to itself include reflections, rotations, and translations. Non-Isometries of $\mathbb{E}^2$ to itself include projection onto a line, shearing, and scaling since none of these preserve distance between points. Consider as well the map from $\mathbb{R}$ to $\mathbb{E}^2$ defined by $x \rightarrow (x,0).$ While this map is an isometry, the two spaces are not isometric since the mapping is not surjective and no such surjective mapping exists. While it “feels” right that the real number line and the Euclidean plane are not isometric (they have different dimensions for starters), actually showing that they are not isometric is much more difficult and beyond the scope of this blog post.

Our overall goal is to define some sort of relationship so that a group $G$ would be “equivalent” to its Cayley graph. How to go about doing this is not immediately obvious, since $G$ is a group that does not depend on the choice of generating set and the Cayley graph is a graph that does depend on the choice of generating set. In order to do this we will massage the definition of an isometry and define a quasi-isometry and what it means for two spaces to be quasi-isometric. This is a common practice in math: if a definition isn’t working the way you want it to, loosen the definition slightly and hope that you aren’t loosening it so much that it is no longer useful.

Quasi-Isometries

Many of the spaces we will be interested in studying will be graphs, thus it will be helpful to define a metric for graphs before diving into the heart of quasi-isometries.

Let $\Gamma$ be a graph and let us induce a metric on $\Gamma$ by path length. We can think of each edge as an isomorphic copy of the interval $[0,1]$ . We want to think of the edges like this so that we can define any point between two vertices. For any given path between two vertices, we will set the starting vertex as zero and then build the path up, with each vertex we pass adding a total of one to the path and following edges only partially resulting in adding the corresponding fraction of one to the path. We then define the metric $d_\Gamma(x,y)$ to be the infimum of the set of path lengths of all possible paths between $x$ and $y$ . Checking that $d_\Gamma(x,y)$ is in fact a metric is straightforward and left as an exercise to the reader. Let us now formally define path.

Definition. A path of length $L$ is an isometric embedding of $[0,L]$ on $X$ .

The paths we will care most about are those of minimum length:

Definition. A geodesic is a path of minimum length. Additionally, a metric space is called geodesic if all pairs of points can be connected with a geodesic.

For example, $\mathbb{E}^2$ and $\Gamma$ with the path metric are both geodesic. However, if we remove the origin from $\mathbb{E}$ we no longer have a geodesic. Consider a point on the negative x-axis and a point on the positive x-axis. In order to connect them with a path of minimum length we would need to go through the origin. Given any path that went around the origin, we would always be able to find a shorter path that got closer to the origin and would thus be shorter. Hence, the space is not geodesic.

We have now formally established graphs as metric spaces and can consider their isometries. Recall that our goal was to find some sort of equivalence between a group and its Cayley graph (with respect to some generating set). We know that this relationship cannot simply be being isometric, since $G$ is always countable and $\Gamma_{G,S}$ is always uncountable (every edge is isometric to $[0,1]$ which is itself uncountable). Thus, there will be no surjective mapping from $G$ to $\Gamma_{G,S}$ . We will fix this problem by loosening our definition of isometry, beginning with the subjectivity requirement.

Definition. A net in a metric space $X$ is a subset $P \subseteq X$ such that $N_R(P)=X$ for some $R\ge 0$ and $R$ being finite.

Essentially, a net is a subset of points in the metric space which are spread out enough around the space, so that if we blur the edges of the net (take some finite neighborhood around each point in $P$ ), we get the whole set $X$ . Hence, it doesn’t make sense to talk about the net of a finite set, since we can simply pick any point $x\in X$ that pleases us and then set $R$ to be large enough to cover the whole set $X$ .

The idea of a net allows us to define a mapping $f$ from $G$ to $\Gamma_{G,S}$ where $f(G)$ is the set of vertices of the Cayley graph and thus $f(G)$ is a net is $\Gamma_{G,S}$ . However, now we don’t have inverses. We fix this by loosening our definition in a slightly different way:

$d_x(x,y)-\epsilon \le d_y(f(x),f(y)) \le d_x(x,y) + \epsilon$ .

This statement says that the distance under the mapping is “close enough” to the distance in the original metric space where there is some sort of fudge factor which works for all pairs of points $x,y$ .

Finally, using this additive fudge factor and adding in a multiplicative fudge factor to account for variations between generating sets of groups, we get our definition of quasi-isometric and quasi-isometry.

Definition. If $X,Y$ are metric spaces, $f: X\rightarrow Y$ is $(K,\epsilon)-$ quasi-isometric if

$\frac{1}{K} d_x(x,y) - \epsilon \le d_y(f(x),f(y)) \le Kd_x(x,y)+\epsilon$ .

Furthermore, if $f(X)$ is a net in $Y$ , then $f$ is a quasi-isometry.

Notice that this definition doesn’t quite mirror the definition of isometry/isometric. In fact, the distance-preserving relationship falls under quasi-isometric and isometry and the additional requirement of being surjective-(ish) falls under being isometric and being a quasi-isometry.

This achieves our goal! There is a quasi-isometry between a group and its Cayley graph, and two Cayley graphs of the same group are quasi-isometric. Next time we will show that being quasi-isometric is an equivalence relation on metric spaces.

Sources:

https://en.wikipedia.org/wiki/Random_group

https://en.wikipedia.org/wiki/Hyperbolic_group

2 Responses to Week 7 Monday: Hyperbolic Groups and Quasi-Isometry

Akash Ganguly says:

October 31, 2022 at 3:20 am

Great post Michaela! As MurphyKate mentioned, we’ve decided to skip some backgrounding on hyperbolic geometry, but since my final project topic is something to do with it, I have some fun facts and sources to share. Fun fact! There are no squares in hyperbolic geometry. There is a ‘biggest’ triangle in hyperbolic geometry!

I’ve linked a short video I thought was cool

https://www.youtube.com/watch?v=zQo_S3yNa2w

Osip Surdutovich says:

November 3, 2022 at 7:14 am

Great post Michaela, really clear! It’s intriguing how we allow ourselves to squint our eyes at all these structures in order to make the theorem work. From one hand, it seems a bit unscientific, but it also works and helps us describe very valuable ideas. I guess this is also how physics/chemistry work with classical and quantum scales embedded in the science. I’ll think about this some more.

Hyperbolic Groups

Isometries

Quasi-Isometries

2 Responses to Week 7 Monday: Hyperbolic Groups and Quasi-Isometry

Leave a Reply Cancel reply

Recent Posts

Recent Comments