Week 5 Monday : Serre’s Property FA and Normal Forms

Serre’s Property FA

Welcome to Week 5! I can believe we’re halfway done with this class! We ended Wednesday’s class on free products with a claim : if and is finite, then is conjugate to a subgroup of or .

It turns out that this is actually a corollary of a much more general statement, albeit one that at first sight appears to be talking about something completely separate. We want to show that every finite group under any action on any tree has a universal fixed point. I’ll provide a somewhat sketchy proof below; you’ll see that it learns heavily on the finiteness of the group.

Pick an arbitrary tree and an arbitrary action. Let’s now pick an arbitrary vertex and look at the orbit of that vertex under that action. Since our group is finite, this orbit will be finite too. Let be the subtree spanned by the orbit of this vertex. Since our orbit is finite, this subtree will be too! So then acts on . Note that it will act on the vertices in our orbit, but the intermediate vertices will be acted on as well because there is a unique path between two vertices, so under the action this path must be preserved, so these intermediate points all must be translated too. This action preserves degree! This allows us to ‘prune’ the leaves (vertices with degree one). If we continually prune this subtree, we are left with two cases.

In the first case, if it acts on a singular vertex, this action must be the trivial action! In the other case, either one of the vertices is the fixed vertex or the midpoint of the edge is a the fixed vertex. Boom.

This property — that every action by a group G on any tree leaves a global fixed point — is called Serre’s Property Fixe Arbre (Property FA) for short. Now back to our original claim : if and is finite, then is conjugate to a subgroup of or . We have shown that every finite group has Property FA, so let us consider a tree acts on: the Bass-Serre tree! Recall that stabilizers of edges of the Bass-Serre tree are trivial, so the only case is that the universal fixed point can be a vertex, whose stabilizer is conjugate to or in our Bass-Serre tree. Boom.

This is where we say goodbye to free groups (at least as one of our primary objects of study), so I’ll state some cool theorems about Property FA (without proof, check out section 3.10 in Groups, Graphs, and Trees for proofs).

If has Property FA, then any quotient of G also has Property FA.

For has Property FA.

The automorphism group of a free group where has Property FA.

I think it’s cool to think about the automorphism group of a free group because first of all it’s an example of an infinite group (this group is huge!) with property FA and also it highlights another link free groups have with linear algebra! Jakob Nielsen showed that the automorphism group of the free group with basis is generated by the 4 elementary Nielsen transformations which are

1. swapping two generators
2. cyclically permuting the whole set of generators
3. replacing a generator with its inverse
4. replacing a generator 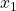with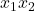
   
   These 4 transformations correspond to the elementary row operations! The first two are the operations of switching any rows you’d like. The third is scaling a row by an invertible scalar, and the fourth corresponds to adding rows.
   

Normal Forms

We now switch our focus to the word problem, except this time more in depth. To do so we introduce some vocabulary and review some notions from before. Let’s start with an example we’re familiar with : a presentation for . Take a look at two elements on this generating set, and . According to our presentation, these elements should refer to the same thing, but they’re spelled differently. This is exactly to do with the word problem – it’s a question of how we define these equivalence classes.

Let’s take a step back. Take a generating set , our alphabet and consider the free monoid which is words over our alphabet, containing formal inverses. Note the subtlety — contains formal inverses hinting at the fact that we’ll later want to consider this as a group, but we haven’t put any relations in place between these formal inverses yet! Then, there is a natural transformation . Note that placing this group structure gives meaning to elements in our free monoid, but our problem with the mispellings of the same still has not been solved.

Ideally, we’d like one clean representative on our alphabet per element of our group . This is exactly the idea of a normal form. It is a choice of name for each element in . More formally, it is a function such that .

Let’s look at a few examples.

Our old friend the free group

The most logical normal form might be freely reduced words, but another possible normal form (courtesy of Horace) is where is freely reduced. This example highlights the fact that a normal form is a choice! Even though this is a super weird choice, it is still a normal form.

Let’s now look at

Here, we get a ton of different options. We can move all of one generator to the front, or the other, or some mix.

Since we’re more comfortable with , let’s pick a word on our alphabet, say look at the normal forms on our Cayley graph.

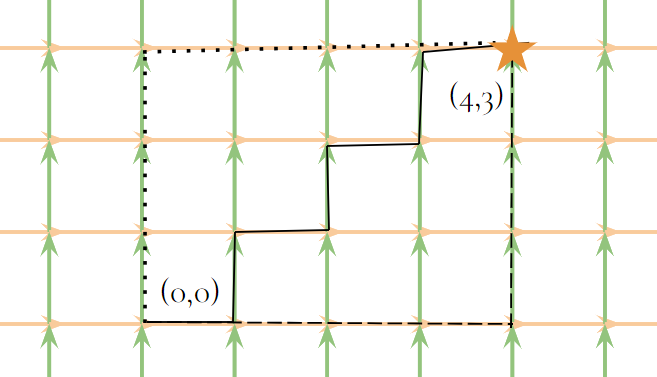
Taking the green arrows to be our generator and the orange to be our generator , we can see that normal forms correspond to picking paths to elements in our Cayley graph! Under , our word looks like and in the graph, this means go all the way up, then all the way right. Under , it is , that is, go all the way right, then all the way up. Finally, our funkiest one makes our word , which says take steps up, then go all the way right.

We also talked about normal forms that were ‘combings’, that is any path to an element that is already on a larger path to a different element is also a normal form. In other words there are no annoying knots (backtracks for example) when we choose an element on a path and inspect its normal form.

The idea of normal forms transforms our word problem — we ask if given a word on our generators, can we find the normal form for this word in a finite amount of time? As Sam pointed out, this is really just transferring of our algorithm.

Next class we’ll build up some more vocabulary to talk more concretely about the word problem and machines we’ll build to potentially solve it. Thank you for reading!